There are two basic types of errors that can occur in hypothesis testing:

• Type A or 1 Error: The null hypothesis is correct but is incorrectly rejected.
• Type B or 2 Error: The null hypothesis is incorrect but is not rejected.

The traditional way of explaining testing errors is with a table like the one shown below:

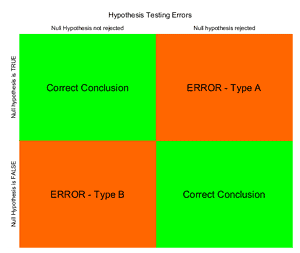
Typically, we’re more worried about Type A errors than Type B–rejecting a hypothesis that is not actually incorrect.

The chance of making a Type A error is referred to as the alpha risk or alpha level; the chance of making a Type B error is referred to as the beta risk or beta level.

Need more explanation? Khan Academy’s video does a good job of walking through Type A (or Type 1) errors:

ASQ Six Sigma Green Belt Errors in Hypothesis Testing Questions:

Question: When an inspection process rejects a conforming product, what type of error is being made?

(A) a
(B) b
(C) σ
(D) H0

Answer:

A: 0.05 – Remember that alpha risk is the risk that we have incorrectly rejected the null hypothesis even though it is correct, one of the kinds of errors we can make. 0.05 alpha means that we are comfortable with a 5% chance of incorrectly rejecting the null. That is a common industry standard. Even if you didn’t know that the other answers don’t make sense. At 50%, you are basically flipping a coin! Also, see the guide to hypothesis testing.